3.1 «Classical» Optical Microscopy

Basic definitions.

The term used in a title is rather uncertain. In microscopy the recent progress of optical methods resulted in the appearance of a large number of techniques many of which can be considered as classical. However, the purpose of this chapter is not to review the wide capabilities of optical microscopy but define some terms which will be used further for the explanation of a confocal microscope principle of operation. We introduce these basic concepts taking the widefield optical microscope as an example (Fig. 1).

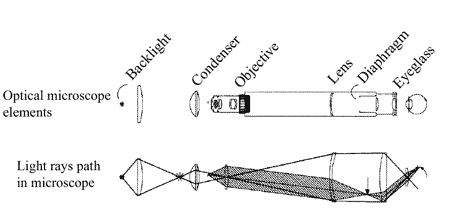
Fig. 1. "Classical" optical microscope schematic diagram.

In such a microscope, the image of an object placed in a uniformly illuminated field of view is projected by an optical system onto the retina of the eye or onto a sensor (e.g. CCD array in a video camera) plane. Generally, a sensor receives the light emitted by different areas of a specimen which are both in a focal point of an objective lens and beyond a focal point (Fig. 2).

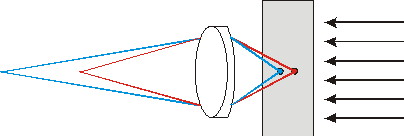
Fig. 2. In a widefield microscope, various points of an object are viewed simultaneously, therefore points of planes, other than the object plane, produce background illumination lowering the contrast.

Numeric aperture and dimensionless units.

To describe parameters of optical instruments, some special terms are adopted in optics. Namely, numeric aperture is defined as

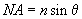
(1)

where – refractive index of a media, – half-angle of a cone within which light rays converge or diverge. For a lens, this angle is defined by its diameter and focal length

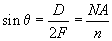
(2)

It is convenient to measure distances from the axis in the object plane by the units of the light wavelength in the media , where – light wavelength in vacuum. Dimensionless radius unit in this case will be written as

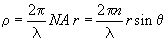
(3)

while dimensionless distance along the optical axis will be

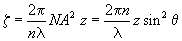
(4)

What factors determine a microscope resolution?

Images are formed by lenses or mirrors in geometrically conjugate planes. In this case, for rays emanating from every point of the object, the Fraunhofer diffraction condition is met. Let, for example, a parallel beam from the distant point object converge in a lens focal plane (Fig. 3). Each point in the focal plane corresponds to the point at infinity, therefore, the Fraunhofer diffraction condition is met in the focal plane. Diaphragm which confines the beam plays the role of an obstacle for light diffraction. Such a diaphragm, in particular, can be the lens mount. This is the case of the diffraction at the optical system entrance aperture.

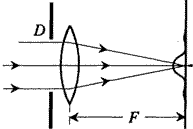
Fig. 3. Fraunhofer diffraction in a lens focal plane.

Similarly can be considered the case when the point object is positioned at a finite distance from the lens and the image is formed at a distance from the lens on its right-hand side. Distances and obey the lens formula:

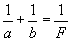
(5)

To explain why Fraunhofer diffraction takes place in this case also, we replace the single lens with focal length by two closely situated lenses with focal lengths and (Fig. 4). Then the source will be positioned in the front focal point of the first lens and the image plane coincides with the rear focal plane of the second lens. Condition (1) is automatically met in this case because it is equivalent to the optical power (i.e. inverse of a focal length) sum rule of two closely situated lenses. Between the two lenses light rays travel as a parallel beam. Comparing Fig. 3 and 4 it can be concluded that in the second case the Fraunhofer diffraction occurs at the common lens mount and is viewed in the rear focal plane of the second lens. Fig. 3 corresponds to the diffraction pattern in the telescope objective (or the eye), Fig. 4 – to the light diffraction pattern in the microscope objective. Field of view of conventional microscopes does not exceed 1000 resolved picture elements.

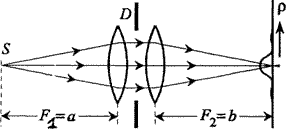
Fig. 4. Fraunhofer diffraction in a plane geometrically conjugate with a source.

The point spreading function (or the function of the diffraction-limited system pulse response) determines the intensity distribution in the lens focal plane due to the Fraunhofer diffraction from the entrance aperture. As it was shown before, exactly the same intensity distribution from a point source is formed in the conjugate plane of a thin lens.

PSF of the light beam limited by a circular aperture with diameter for the lens having focal length can be expressed in a general form as follows [2]:

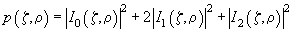
(6)

where

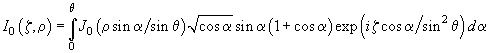
(7)

where – k-th order Bessel functions, .

Here we introduce the more general function as compared with that given before. Function gives intensity distribution along radius for different planes . This function has a remarkable property for any plane :

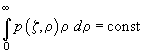
(8)

which means that energy flux through every plane is constant.

In the paraxial approximation (small magnitudes), the light intensity distribution in the focal plane is given by:

(9)

where the normalization coefficient is selected so that value in a focal point is equal to 1.

The diffraction pattern from a circular aperture is concentric rings. A central bright spot is called the Airy disk. The first bright ring maximum intensity is about 2% of the intensity in the center of the Airy disk. Distribution is shown in Fig. 5.

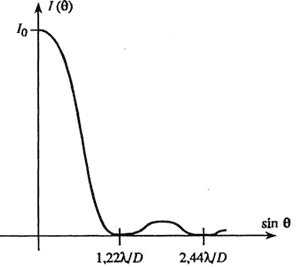
Fig. 5. Intensity distribution of light diffracted by a circular aperture.

The Airy disk radius is:

(10)

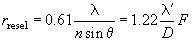
(11)

where .

It should be noted that on the system optical axis : and , therefore the resolution along the optical axis is determined only by contribution of . In the paraxial approximation (small magnitudes), the relative intensity distribution along the axis is given by:

(12)

Microscope resolution, Rayleigh criterion.

Resolution of the microscope generally means the capability to distinguish two point objects of about equal intensity. From the function of intensity distribution in a focal plane it follows that the resolution is determined by overlapping of Airy disks of two point-like objects. Rayleigh proposed the criterion which states that two points are resolved if a "dip" in their images intensity is 26% of the maximum intensity. Also, the separation distance between two resolved points should be more than the Airy disk radius (see previous paragraph).

Summary.

The main characteristic of the objective lens is its numeric aperture determined by its diameter and a focal length.
Resolution of a conventional optical microscope is determined by the Fraunhofer diffraction at the entrance aperture of the objective lens. The minimum distance between resolved point objects of equal intensity amounts to the Airy disk radius.
In present chapter, the expression for the point spreading function (or the function of the diffraction-limited system pulse response) is derived which will be used further for the explanation of the confocal microscope operation.

References.

Robert H. Webb, "Confocal optical microscopy" Rep. Prog. Phys. 59 (1996) 427-471.
Richards B. and Wolf E., "Electromagnetic diffraction in optical systems II. Structure of the image field in an aplanatic system" Proc. R. Soc. A 253 (1959) 358-379.