2.7.1 MFM general concept
The magnetic force microscopy general concept is the registration of the force interaction between a magnetic probe and a sample's magnetic field. Today, there are two modes of the MFM operation: static MFM [1, 2] and dynamic MFM [3, 4].
In the static MFM mode (dc), the interaction force
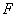is measured through the detection of the cantilever deflection d from the equilibrium position which is given by
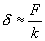
(1)
where k – the cantilever spring constant (see chapter 2.1.2).
In the dynamic MFM mode (ac), the change in resonant properties of the vibrating system cantilever-sample is registered. In this case the amplitude
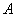, phase
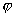and resonant frequency
of the cantilever oscillation must be detected. If the force with the gradient
acts on the cantilever in the vibration direction z, the above mentioned parameters variation can be expressed as
(2)
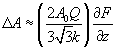
(3)
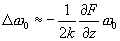
(4)
where k – cantilever spring constant, Q – vibrating system quality factor, А0 – amplitude of cantilever oscillation at resonant frequency
in the absence of external force gradient (see chapter 2.3.4). As can be seen from (2)–(4), all three experimentally determined parameters are linear functions of the force derivative. In practice, however,
is mainly determined by measuring the cantilever phase variation (2).
Let us estimate forces and their gradients which can be detected in the MFM. To do that, we calculate the interaction force between two point magnetic moments m1 and m2 separated by distance r. Suppose one particle is on the cantilever tip and the other - on the sample surface. The field created by m1 at m2 location is:
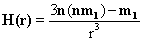
(5)
where n – unit vector in the r direction. The tip will experience the force which is defined as the energy gradient:
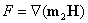
(6)
where B – magnetic field from the sample. If m2 is independent of coordinates, expression (6) can be rewritten as:
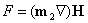
(7)
Assuming that dipoles m1 and m2 are oriented along the Z-axis, the force of their interaction in accordance with (5), (7) is F = –6m1m2/z4 and force gradient is
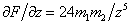. For iron (Fe) particles having diameter 100 Å magnetized to saturation (m = 10–16 emu) and separated by distance 100 Å the resultant force is estimated as ~10–11 N and force gradient is ~10–2 N/m. According to expression (1), deflection of the silicon cantilever beam with spring constant
(
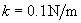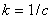is calculated using cantilever dimension and Young's modulus, see (12) in chapter 2.1.2) under the point force
is equal to
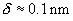. This value corresponds to the minimal detected deflection of modern AFM cantilevers.
The force gradient
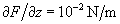results in the phase shift of the cantilever with quality factor Q = 200 and spring constant
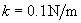equal to
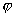which is much more than the minimal detected signal.
Summary.
- Two modes of the tip-sample magnetic interaction exist: static MFM (dc) and dynamic MFM (ac).
- In the dc mode the interaction force is measured while in the ac mode - the force gradient.
- Dynamic mode of MFM operation is more sensitive to the magnetic field variation than the static one.
- In both modes the detected signals do not depend directly on the sample magnetic properties. Therefore, it is necessary to consider available algorithms of the quantitative interpretation of MFM data (see chapter 2.7.2).
References.
- Appl. Phys. Lett. 50, 1455 (1987).
- J. Appl. Phys. 62, 4293 (1987).
- P. Grutter, H.J. Mamin, D. Rugar, in Scanning Tunneling Microscopy II, edited by R. Wiesendanger and H.-J. Guntherodt (Springer, Berlin, 1992) pp. 151-207.
- S. Manalis, K. Babcock, J. Massie, V. Elings, M. Dugas, Appl. Phys. Lett. 66, 2585 (1995).